Explanation:
Typical applications to the interrelations given above are in manometer problems. A typical manometer could be exposed to different pressures at it's openings and is filled with one or more different fluids. A typical manometer problem could as you to find the pressure drop across the two openings. Here, given the following diagram, we derive an expression for the pressure drop.
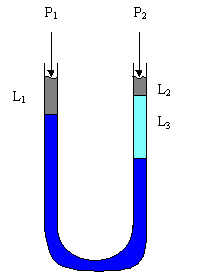
Here, LHS and RHS represent the left and right hand side of the manometer. We begin with writing the pressure representation of each component, then we gradually simplify the expression to get it into a reduced form.
or
or, with heights known, and LT representing the total length of the manometer, we get,
now, we can rearrange the equation, with the total pressure drop written as DP
or,
This is our expression, given or looking up the quantities on the right side of the equation allows for a determination of the pressure drop.
Example 2:
The great Boston molasses flood occurred on January 15, 1919. In it, 2.3 million gallons of crude molasses flowed from a 30-foot high storage tank that ruptured, killing 21 people and injuring 150. The estimated specific gravity of crude molasses is 1.4 (so the density of the molasses was 1.4 g/ml). What were the mass of molasses in the tank in lbm and the pressure at the bottom of the tank in lbf/in.2?
Example 3:
PLACE "PRESSURE BMP" HERE. A fluid of unknown density is used in two manometers-one sealed-end, the other across an orifice in a water pipeline. The readings shown here are obtained on a day when barometric pressure is 756 mm Hg. What is the pressure drop accross the pipeline manometer?
