So you now know how to identify if a gas-liquid system has a single condesible species. But, what are the interrelationships that will help us?
There are four saturation formulas that apply to gas-liquid systems that have only a single condensible species, and they are listed here:
| Relative Saturation |  |
|---|---|
| Molal Saturation | 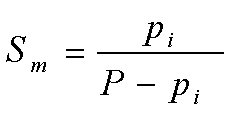 |
| Absolute Saturation | 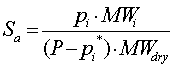 |
| Percentage Saturation | 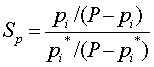 |
P is the Pressure of the total gas mixture
pi is the Partial Pressure of component i
pi* is the Vapor Pressure of component i
MWi is the molecular weight of component i
MWdry is the molecular weight of total gas mixture
There is special notation and terminology that we use for air-water systems since they are so common. However, the term saturation in each of these equations is designated for any generic gas-vapor system: when the gas is air and the vapor is water vapor, then the word saturation is replaced with humidity for air-water systems.
Take the first equation for example:
The name "relative saturation" changes to "relative humidity". When we look at sr, it changes to hr, and both pi and pi* specifically refer to water. This means the pressure could be written as pH2O and pH2O*.
The formulas stay the same whether we are talking about any gas-vapor system or specifically an air-water system; there is just a slight change in terminology and notation. The most important thing to remember is that if a problem statement gives or asks for one of these quantities, you now know where to look them up and how to use them.
Example 1
A gas consists of 10% styrene and 90% air in a tank at 100 degrees Celcius and 1000 mmHg. Calculate the relative saturation, molal saturation, and percentage saturation.
Example 2
Water enters a boiler at 80 degrees Celcius under atmospheric pressure. If its relative humidity is 60%, what is the vapor pressure, partial pressure, and mole fraction of water in the air.
