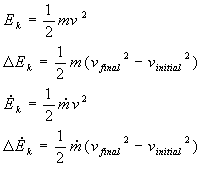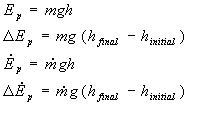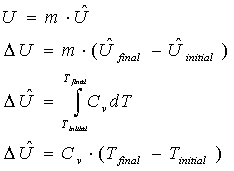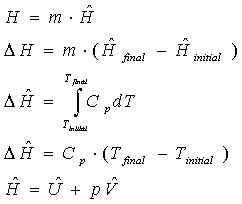Energy Balance Intro

Upon studying this section, you should be familiar with the following:

This section gives the tools needed to do Energy Balances

Explanation:

This section prepares us for energy balances, much as we did for material balances. If you recall, material balances where not performed until chapter four. Before we could jump into doing material balances, we covered topics on units, pressure, temperature, and flow rates, to name a few. We were not prepared to solve material balance problems until we understood a few basic concepts and definitions.

Similarly, this section introduces terminology, fundamental definitions and mathematical relationships required to perfom energy balances.

Types of Energy

Explanation:

There are really three types of energy that we'll deal with often in chemical engineering: Kinetic Energy, Ek, Potential Energy, Ep, and Internal Energy, U. Closely related to Internal Energy is Enthalpy, H. Now, lets introduce the definitions, mathematical descriptions, and the concepts for each type of energy.

Kinetic Energy

  • Definition: Energy due to motion

  • Relation:

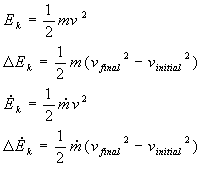  • Where m is for mass, v is for velocity, and the dot signifies a rate.

  • Examples: A moving car, a gas molecule, and a flowing stream all have kinetic energy. A parked car, a molecule in a solid lattice, and a stationary glass of water are all systems that do not possess kinetic energy (because they do not have a non-zero velocity).

  • Note: If the system is not accelerating or decelerating (changing velocity), DEK = 0. That is, the system has kinetic energy, but that energy doesn't change as you move between two point unless the system has undergone a change in velocity: the change in kinetic energy is zero. In the bulk of energy problems that you will deal with, that is, those in which there is a chemical reaction, a change of state, or substantial temperature change, the change in kinetic energy is very small (DEk = 0).

    • Potential Energy

  • Definition: Energy due to position

  • Relation:

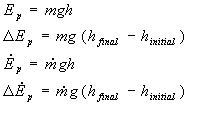  • Where m is for mass, g is gravity, h is a height, and the dot signifies a rate

  • Examples: A bungee jumper standing on the edge of a cliff, a book resting on a table, a jacket hung in a closet, or anything that is above the ground possesses potential energy due to gravity. So, someone standing on the ground, and shoes that rest on the ground are both systems that possess no potential energy due to gravity.

  • Note: If the system neither rises nor falls, the change in potential energy is zero (DEp = 0). Additionally, rarely does the system rise or fall substantially in the energy balance problems that you will perform for chemical engineering problems in this class, so you will find that the potential energy change is often negligable and set to zero. Finally, you will notice that you will only deal with potential energy due to gravity in this course. If any other source of potential energy relavent, such as electrical potential, or some other type, that will be clearly stated in the problem statement.

    • Internal Energy

  • Definition: "molecular energy", due to molecular interactions

  • Use: in energy balances on closed systems

  • Relation:

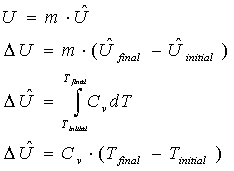
      • CV = the heat capacity at constant volume.

    • Here, there are two equations to find the specific internal energy using a term with a hat over it (U-hat); the third equation is used if the heat capacity is a function of temperature (often for gases). The last equation is for a constant heat capacity, (often used for liquids and solids). Each of these equations involves the use of specific properties (those with hats over them, meaning that they are "per mol" or "per mass". Once we find the specific internal energy change with the third or fourth equations, we can find the internal energy change by multiplying it by the amount of substance undergoing that change. Additionally, we can find the rate of internal energy change (DU-dot) by multiplying by the mass or molar flow rate.

    It is assumed that U-hat has units of Energy/mass in the above equations, when the first formulas could be used. If U-hat has units of Energy/mole, of course, we would multiply the specific internal energy by moles (and not mass) to get the internal energy (using, for example, U = n * U-hat). It may be more correct to write U = (n or m) * U-hat. The key to getting this write is to pay attention to the units of the specific property. This of course applies to all specific properties (U-hat, H-hat, V-hat, etc.) and is probably a point that you are already familiar with.

  • Example:
    Temperature Change of a Solid
    Imagine a molecule, say a water molecule, is stuck in a lattice (an ice cube). Here, it is able to vibrate a little, but it is fixed in position with respect to its neighboring water molecules. If we neither increase nor decrease the temperature of the freezer (no temperature change) its internal energy ("molecular energy") doesn't change; it simply continues to vibrate to the same extent. INow, if we make the system colder, thes molecule vibrates less, and there is a change in internal energy (a decrease). If we take the ice cube out of the freezer and place it where it is warmer, the molecule vibrates more (gaining energy, an increase in internal energy). If it melts, it further gains internal energy. In this example there is a temperature change to accompany the molecule's change in internal energy.

  • Note: Changes in internal energy occur with processes that change its molecular energy. Thus, internal energy changes occur with a change in temperature (effects a molecule's motion), a chemical reaction (breaking and making bonds), a change of state (change in intermolecular energy), or a substantial pressure change(under high pressures, our H20 molecule in our ice cube vibrates less).

    • Enthalpy

  • Definition: "molecular energy", due to molecular interactions

  • Use: in energy balances on open systems

  • Relation:

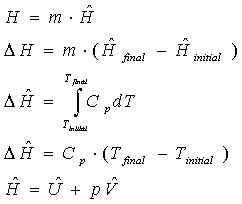
      • Cp = the heat capacity at constant pressure.

    Here, the usage of the first -> fourth equations is analogous to scenarios for those equations for internal energy already explained above. However, the last equations describe the relationship between enthalpy and internal energy. Similar discussions given above in the internal energy section about flow rates and specific properties are applicable here.

  • Example: Enthalpy can be thought of as the "molecular energy," or the internal energy for moving fluids. Thus, if a flow of water in a pipe experiences a change in temperature, it is accompanied by a change in enthalpy.

  • Note: A system experiences a change in enthalpy if there is a change in temperature, a substantial change in pressure, a chemical reaction, or if there is a change in state.

  • Note: Additionally, there is a relationship between the heat capacity at constant volume and the heat capacity at constant pressure, this relation is deferred until later.

    		•

    2 Types of Energy Transfer

    Explanation:

    There are two types of energy transfer, heat and work. There should be a clear distinction between these two types of energy transfer and the forms of energy just mentioned. Where kinetic, potential, and internal energy are all forms of energy, heat and work are energy vehicles that move energy from one place to another. They act to transport energy by carrying energy from the surroundings into the system or from the system to the surroundings. Further examples follow, but let me give you one now: First, we need to name the system and the surrounding. The system could be a pot of water, and let's say that it experiences an increase in temperature (an increase in internal energy). The system gets energy from the surroundings and we say that this energy is transported by heat.

    Heat

  • Definition: Energy transported due to a difference in temperature between the system and the surrounding.

  • Notation:

    where the first notation designates some quantity of heat transported, and the second tells how fast heat is being transported per time.

  • Example: Transport of energy from a stove burner to the pot, from the human body to the air, or from a chemical reaction to a flask is done by heat.

  • Sign convention: "+Q for heat into the system". In otherwords, heat into the system is positive. If a pot of water is the system and the temperature is being increased due to heating, the heat is positive (+Q). If we then let the pot sit and cool, it looses heat to the surrounding, thus a negative heat (-Q).

  • Note: The words adiabatic and insulated are used to describe a system in which heat transfer is zero (Q = 0).

    • Work

  • Definition: Energy transport due to any mechanism other than heat (by a piston, propeller, electric current, electromagnetic radiation, etc).

  • Notation:

    where the first expression designates the general work expression (units, Joule), the second designates work per time (units, Joule/second), and the third is the modified work expression that we will use for open systems, where the s subscript designates shaft work, such as work by a propeller (units, Joule/second).

  • Example: Energy transport due to work when stirring a cup of water increases its temperature, when irradiating a cup of water with microwaves, thus increasing its temperature, or the hammering of a nail, resulting in a warmed metal nail.

  • Sign Convention: Work done on the system is positive (stirring our cup of water), work done by the system is negative (if our system is the computer screen, it releases work to the surroundings in the form of electromagnetic waves).

    • The 1st Law of Thermodynamics

    Explanation:

    "Energy is neither created nor destroyed." There are several other ways to say this, such as the energy of the universe is constant, or that energy doesn't appear spontaneously. That is, our cup of water doesn't increase its temperature sponaneously, but due to energy brought in from the surroundings via heat or work.

    This statement is what allows us to make the energy balances. Energy, like mass, is conserved.

    Getting to Specific Properties

    The last topic has to do with the way properties are given. In earlier sections, we determined that m stood for mass, and mdot stood for mass flow rate. Here, we can determine that V stands for volume, or Vhat, stands for specific volume, volume per amount (mass or mole). The following discussion leads to ways of differentiating between quantities such as volume and specific volume. First we introduce the two following definitions.

    Intensive properties: properties independent of the amount of material.

    Extensive properties: properties dependent on the amount of material.

    Explanation:

    Let me offer you a way to determine whether a property is an external or internal property - If the answer to the following question is yes, then the property is external (otherwise, it is an internal property): Does the property change if we go from one gram to a 100 grams for the substance?

    for example, determine whether the following properties are external or internal. mass (kg), temperature (K), volume (gallons), energy (joules), density (g/mL). The extensive properties listed here (those that change when we change the amount of substance) are: mass, volume, and energy.

    Now we understand how to categorize a certain property as extensive or intensive. Finally, note that any extensive property can be turned into an intensive property if it is divided by an amount. The resulting intensive property is called a specific property.

    For example, mass is an extensive property. If we divide mass by moles, we get an intensive property, namely, the molecular weight of a substance. We can divide an extensive property by either mass or an amount (such as moles) to turn it into an intensive property.

    The reason intensive and extensive properties are covered is to introduce specific properties because we will use them so much in energy balances. That is, many of the physical properties taken from the tables in energy balance problems are listed as specific properties.


    Goto | Interrelations Home | Dr. Blowers Home | U of A Home



    The University of Arizona. All copyrights © reserved.

    © 2007 Arizona Board of Regents for The University of Arizona