(chapter 8, heat transfer for a phase change) Example:
Problem Statement:
b) Determine the rate of generation of steam in the boiler (kg/s) from the following flow diagram:
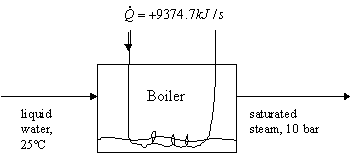
Again, the way we try to approach these problems is to account for all possible consumptions or
generations of energy within the system. Here, we are lucky because we are dealing with water, so
steam tables will make the calculation process particularly easy. If we were given acetone, for
example, determining the enthalpy required for the pressure change would require a non-ideal gas
equation of state. First, we assume that the inlet pressure is 1 atm.
Then, we will calculate the specific enthalpies of the temperature change of water for the inlet
temperature to the boiling point, then get the enthalpy change for the evaporation from literature, and finally we will determine the enthalpy change of the gas from the steam tables.
1)The heat capacity for the inlet stream can be looked up in Appendix B2, where it is given as a polynominal.
So, we apply the following formula,
Find:
1) The specific enthalpy change for the inlet due to a change in temperature, found by evaluating the above integral like:Liquid water
a=.0754, b=0, c=0, d=0, T1 = 298 K, T2 = 373, so,
DĤ 1 = 5.65 kJ/mol
2) The specific enthalpy change for vaporization of water, from literature.
DĤevap = 40.656 kJ/mol
3) The specific enthalpy change from H2O vapor at 100oC and 1 atm to saturated vapor at 10 bar: Note, as can be verified in the appendix, steam at 100oC and 1 atm is saturated. We can go to steam tables and obtain the enthalpies for both of these states to obtain DH for H2 from saturated vapor at 1 atm (about 1 bar) to saturated vapor at 10 bar use:
DĤ 2 = (2776.2 - 2675.4) kJ/kg = 100.8 kJ/kg
Now, we just have to sum all these up to get an expression that will give us the desired flow rate. First, let's get all the specific enthalpies in units of kJ/kg, so we multiply the first two DĤ's by one over the molecular weight, 1/(.018 kg/mole), to give:
DĤ1 = 313.9 kJ/kg
DĤevap = 2259 kJ/kg
and now, we can write an expression for the total enthalpy change of the condenser, with the total heat in give, noting that the mass balance gives mdotH2O,in = mdotH2O,out, which we'll just call mdot, so
9374.7 kJ/s = mdot * (313.9 kJ/kg) + mdot * (2259 kJ/kg) + mdot * (100.8 kJ/kg)
We can solve for mdot, to get mdot = 3.506 kg/s, and this is what we need to find.
