(chapter 8, heat transfer for a phase change) Example:
Problem Statement:
A gas stream consisting of n-hexane in methane is fed to a condenser at 60°C and 1.2 atm. The dew point of the gas (considering hexane as the only condensable component) is 55°C. The gas is cooled to 5°C in the condenser, recovering pure hexane as a liquid. The effluent gas leaves the condenser saturate with hexane at 5°C and 1.1 atm and is fed to a boiler furnace at a rate of 207.4 L/s, where it is burned with 100% excess ari that enters the furnace at 200°C. The stack gas emerges at 400°C and 1 atm and contains no carbon monoxide or unburned hydrocarbons. The heat transferred from the furnace is used to generate saturated steam at 10 bar from liquid water at 25°C.
a) Calculate the mole fracions of hexane in the condenser feed and product gas streanms and the rate of hexane condensation.
b) Calculate the rate at which heat must be transferred from the condenser and the rate of generation of steam in the boiler (kg/s).
Initial Sketch:
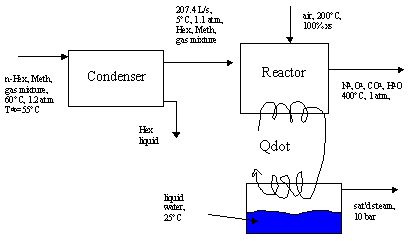
Here, in this section, we are concerned only with the energy balance on the condenser, that is, the problem statement and question in purple. Hence, we're are only concerned with the condenser in the flow diagram. First, we must determine the flow rates of each component into and out of the condenser, thus we first need to do a material balance on the condenser. Thus, we must find part a) above first.
We use the ideal gas law and vapor-liquid equilibrium relations (chapter 6, Raoult's Law) for the material balance on the condenser. This analysis answers part a), and readily gives (to see the condenser balance, click here):

Let's assume that we can approximate that gaseous mixture as ideal, or we can say that they are close enough to 1 atmosphere that we don't have to take into account for the pressure difference from 1 atmosphere. Either way, for the determination of DH around the condenser, we only need to take into account the temperature differences and the phase change.
Now, lets begin the calculation process to get the DH around the reactor. Let's follow this algorithm.1)calculate the DH for temperature changes, for the inlets going to Tb = (68.74 + 273), and for the outlets going from Tb.
2)determine DHhat°condensation
3)add all the inlet, condensation, and outlet DH's to give the total DH around the condenser, which is the heat transferred to the condenser, what we are seeking.
1)The heat capacity for each gas and for the condensate can be looked up in appendix B2, where it is given as a polynominal. This should be programmed into your calculator (Click here to see my program for my ti-89 and a program for the ti-86).
So, we apply the following formula,
The energy exchange with hexane involves a phase change, so we have take the inlet hexane to temperature of the boiling point, where the phase change occurs, and then we have to take the outlet hexane, both the liquid and the vapor, to it's outlet temperature. The only energy exchange with methane is due to a temperature change so we can determine it's enthalpy by considering the temperature of it's inlet and outlet. So now, let's do this:
1) The enthalpy exchange due to changes in temperature. Found by evaluating the above integral.
Methane
a=.01987, b=5.021E-5, c=1.268E-8, d=11.00E-10, T1 = 333 K, T2 = 278, so,
DHhatMe = -1.828 kJ/mol
Hexane
Inlet T to Tb:
a=.13744, b=4.085E-4, c=-2.392E-7, d=5.766E-11, T1 = 333 K, T2 = 341.99, so
DHhatHex,1 = 2.250 kJ/mol
STP to outlet T:
for the leaving vapor, a=.13744, b=4.085E-4, c=-2.392E-7, d=5.766E-11, T1 = 341.99 K, T2 = 278, so
DHhatHex,2 = -15.53 kJ/mol
for the leaving liquid, a=.2163, b=0, c=0, d=0, T1 = 341.99 K, T2 = 278 K, so
DHhatHex,3 = -13.84 kJ/mol
now, we need DH's, so multiply each but their molar flow rate,
DHMe = -1.828 kJ/mol * 9.287 mol/s = -16.98 kJ/s DHHex,1 = +2.250 kJ/mol * 10.46 mol/s = +23.54 kJ/s DHHex,2 = -15.53 kJ/mol * .7044 mol/s= -10.94 kJ/s DHHex,3 = -13.84 kJ/mol * 9.75 mol/s = -134.9 kJ/s
2)
Now, we still have to take into account the energy exchange due to the condensation. Here, we simply look up the value for the condensation of n-Hexane, the only species that experienced a phase change. From the appendix, we readily see:
DHhat’condensation = -28.85 kJ/mol
now, for the enthalpy change, we multiply the specific enthalpy change by the flow rate:
DHdotcondensation = -28.85 kJ/mol * 9.75 mol/s = -281.3 kJ/s
now, we can obtain the total enthalpy change of the condenser, by simply adding up all of the individual enthalpies, which gives us our answer.
DHdotcondenser = ((-16.98)+(23.54)+(-10.94)+(-134.9)+(-281.3))kJ/s = -420 kJ/s
